Perovskites and TMDCs
Novel electronic materials require advanced charge transport modeling and simulation techniques.
For example, perovskite materials are a promising candidate for many semiconductor devices such as lasers, memristors, LEDs and solar cells. Despite being the fastest growing photovoltaic technology, commercialization of perovskite devices faces many challenges which need to be overcome such as degradation of the material. Precise mathematical models and numerical simulations help to advance the technology by understanding how charge carriers move through the device.
Another example are 2D layered transition metal dichalcogenides (TMDCs) such as molybdenum disulfide are promising memristive materials for neuromorphic computing systems as they could solve the problem of the excessively high energy consumption of conventional von Neumann computer architectures. Despite extensive experimental work, the underlying switching mechanisms are still not understood, impeding progress in material and device functionality.
Perovskites and TMDCs share the fact that when simulating charge transport one has to take into account the movement of ions on the crystal lattice. The units cells for both materials are shown in the figure.
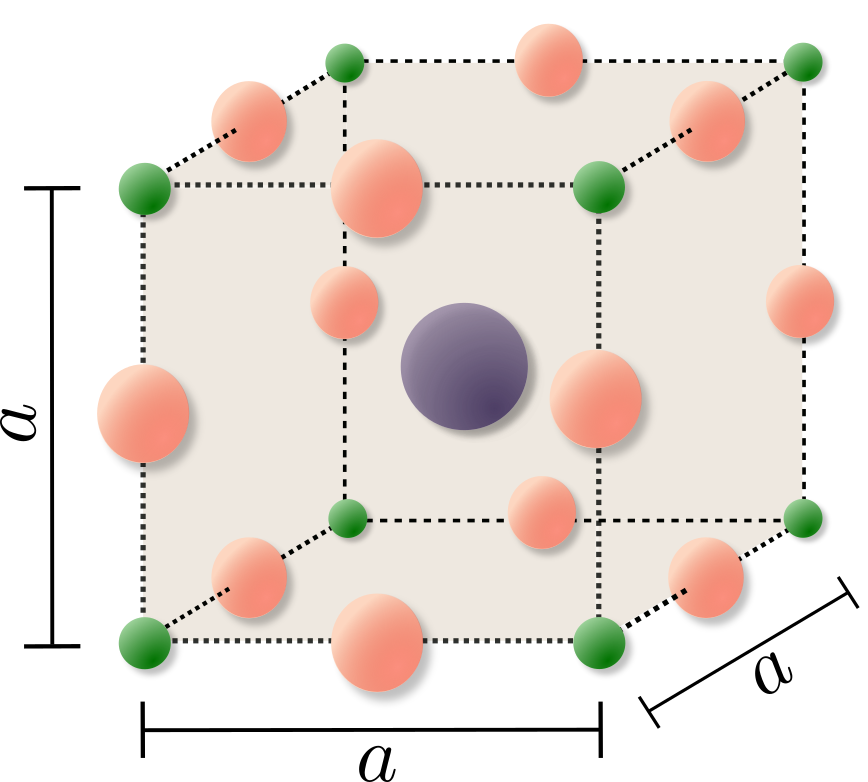
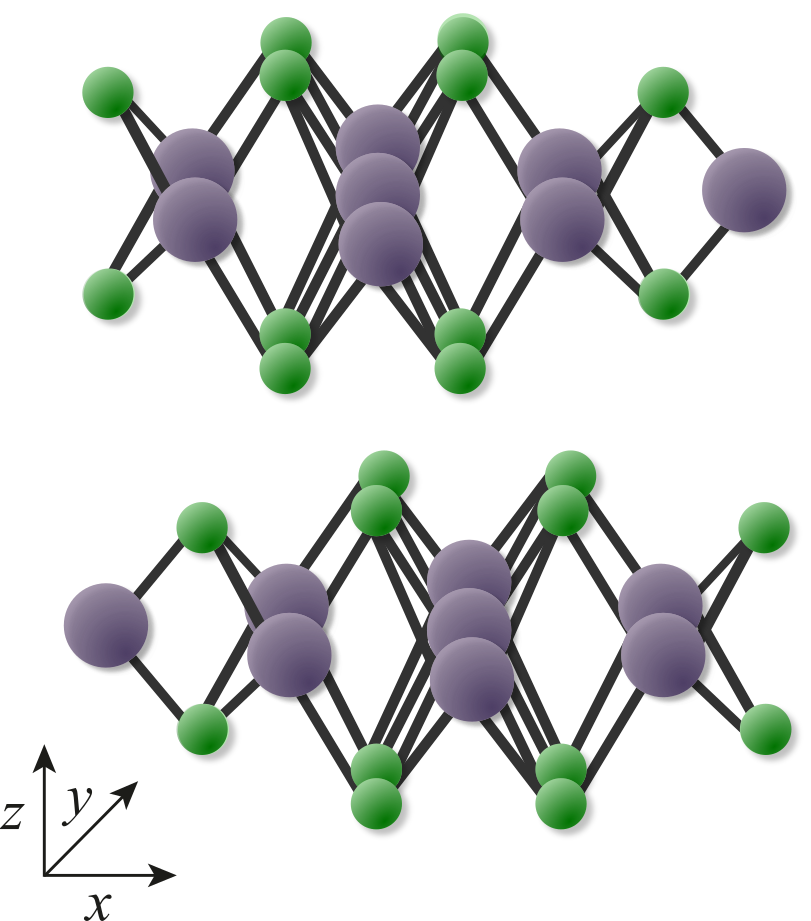
Contribution from the institute
At WIAS, for perovskite materials we derive new potential-based models which take into account an additional ion species as well as corresponding volume exclusion effects on a perovskite lattice. We compare density-dependent diffusion coefficients with density-dependent mobility coefficients and study the existence of numerical solutions. For the simulation we rely on a stable finite volume discretization, as implemented in our flexible 1D/2D/3D Julia tool ChargeTransport.jl. For more details see Abdel et al, 2021, Abdel et al, 2023 and Abdel et al, 2023.
Similar to perovskites, we identified the dominant role of mobile ion vacancies in the switching dynamics of 2D TMDC materials. The switching process is governed by the formation and annihilation dynamics of a local vacancy depletion zone. Moreover, minor changes in the interface potential barriers cause fundamentally different device behavior previously thought to originate from multiple mechanisms. The key mechanisms are identified with a charge transport model for electrons, holes, and ionic point defects, including image-charge-induced Schottky barrier lowering (SBL). The model is validated by comparing simulations to measurements for various 2D MoS2-based devices, strongly corroborating the relevance of vacancies in TMDC devices and offering a new perspective on the switching mechanisms. The insights gained from this study can be used to extend the functional behavior of 2D TMDC memristive devices in future neuromorphic computing applications. For more details see Spetzler et al, 2023.
Publications
 Articles in Refereed Journals
Articles in Refereed Journals
-
D. Abdel, A. Glitzky, M. Liero, Analysis of a drift-diffusion model for perovskite solar cells, Discrete and Continuous Dynamical Systems. Series B. A Journal Bridging Mathematics and Sciences, 30 (2025), pp. 99--131 (appeared online in June 2024), DOI 10.3934/dcdsb.2024081 .
Abstract
This paper deals with the analysis of an instationary drift-diffusion model for perovskite solar cells including Fermi--Dirac statistics for electrons and holes and Blakemore statistics for the mobile ionic vacancies in the perovskite layer. The free energy functional is related to this choice of the statistical relations. Exemplary simulations varying the mobility of the ionic vacancy demonstrate the necessity to include the migration of ionic vacancies in the model frame. To prove the existence of weak solutions, first a problem with regularized state equations and reaction terms on any arbitrarily chosen finite time interval is considered. Its solvability follows from a time discretization argument and passage to the time-continuous limit. Applying Moser iteration techniques, a priori estimates for densities, chemical potentials and the electrostatic potential of its solutions are derived that are independent of the regularization level, which in turn ensure the existence of solutions to the original problem. -
Y. Hadjimichael, Ch. Merdon, M. Liero, P. Farrell, An energy-based finite-strain model for 3D heterostructured materials and its validation by curvature analysis, International Journal for Numerical Methods in Engineering, 125 (2024), pp. e7508/1--e7508/28, DOI 10.1002/nme.7508 .
Abstract
This paper presents a comprehensive study of the intrinsic strain response of 3D het- erostructures arising from lattice mismatch. Combining materials with different lattice constants induces strain, leading to the bending of these heterostructures. We propose a model for nonlinear elastic heterostructures such as bimetallic beams or nanowires that takes into account local prestrain within each distinct material region. The resulting system of partial differential equations (PDEs) in Lagrangian coordinates incorporates a nonlinear strain and a linear stress-strain relationship governed by Hooke?s law. To validate our model, we apply it to bimetallic beams and hexagonal hetero-nanowires and perform numerical simulations using finite element methods (FEM). Our simulations ex- amine how these structures undergo bending under varying material compositions and cross-sectional geometries. In order to assess the fidelity of the model and the accuracy of simulations, we compare the calculated curvature with analytically derived formula- tions. We derive these analytical expressions through an energy-based approach as well as a kinetic framework, adeptly accounting for the lattice constant mismatch present at each compound material of the heterostructures. The outcomes of our study yield valuable insights into the behavior of strained bent heterostructures. This is particularly significant as the strain has the potential to influence the electronic band structure, piezoelectricity, and the dynamics of charge carriers. -
D. Abdel, N.E. Courtier, P. Farrell, Volume exclusion effects in perovskite charge transport modeling, Optical and Quantum Electronics, 55 (2023), pp. 884/1--884/14, DOI 10.1007/s11082-023-05125-9 .
Abstract
Due to their flexible material properties, perovskite materials are a promising candidate for many semiconductor devices such as lasers, memristors, LEDs and solar cells. For example, perovskite-based solar cells have recently become one of the fastest growing photovoltaic technologies. Unfortunately, perovskite devices are far from commercialization due to challenges such as fast degradation. Mathematical models can be used as tools to explain the behavior of such devices, for example drift-diffusion equations portray the ionic and electric motion in perovskites. In this work, we take volume exclusion effects on ion migration within a perovskite crystal lattice into account. This results in the formulation of two different ionic current densities for such a drift-diffusion model -- treating either the mobility or the diffusivity as density-dependent while the other quantity remains constant. The influence of incorporating each current density description into a model for a typical perovskite solar cell configuration is investigated numerically, through simulations performed using two different open source tools. -
D. Abdel, P. Vágner, J. Fuhrmann, P. Farrell, Modelling charge transport in perovskite solar cells: Potential-based and limiting ion depletion, Electrochimica Acta, 390 (2021), pp. 138696/1--138696/12, DOI 10.1016/j.electacta.2021.138696 .
Abstract
From Maxwell--Stefan diffusion and general electrostatics, we derive a drift-diffusion model for charge transport in perovskite solar cells (PSCs) where any ion in the perovskite layer may flexibly be chosen to be mobile or immobile. Unlike other models in the literature, our model is based on quasi Fermi potentials instead of densities. This allows to easily include nonlinear diffusion (based on Fermi--Dirac, Gauss--Fermi or Blakemore statistics for example) as well as limit the ion depletion (via the Fermi--Dirac integral of order-1). The latter will be motivated by a grand-canonical formalism of ideal lattice gas. Furthermore, our model allows to use different statistics for different species. We discuss the thermodynamic equilibrium, electroneutrality as well as generation/recombination. Finally, we present numerical finite volume simulations to underline the importance of limiting ion depletion.
 Contributions to Collected Editions
Contributions to Collected Editions
-
A. Glitzky, On a drift-diffusion model for perovskite solar cells, in: 94th Annual Meeting 2024 of the International Association of Applied Mathematics and Mechanics (GAMM), 24 of Proc. Appl. Math. Mech. (Special Issue), Wiley-VCH Verlag, Weinheim, 2024, pp. e202400017/1--e202400017/8, DOI 10.1002/pamm.202400017 .
Abstract
We introduce a vacancy-assisted charge transport model for perovskite solar cells. This instationary drift-diffusion system describes the motion of electrons, holes, and ionic vacancies and takes into account Fermi--Dirac statistics for electrons and holes and the Fermi--Dirac integral of order -1 for the mobile ionic vacancies in the perovskite. The free energy functional we work with corresponds to that choice of the statistical relations. To verify the existence of weak solutions, we consider a problem with regularized state equations and reaction terms on any arbitrarily chosen finite time interval. We motivate its solvability by time discretization and passage to the time-continuous limit. A priori estimates for the chemical potentials that are independent of the regularization level ensure the existence of solutions to the original problem. These types of estimates rely on Moser iteration techniques and can also be obtained for solutions to the original problem.
 Preprints, Reports, Technical Reports
Preprints, Reports, Technical Reports
-
A. Thayil, L. Ermoneit, M. Kantner, Theory of valley-splitting in Si/SiGe spin-qubits: Interplay of strain, resonances and random alloy disorder, Preprint no. 3158, WIAS, Berlin, 2024, DOI 10.20347/WIAS.PREPRINT.3158 .
PDF (5807 kByte) -
Y. Hadjimichael, O. Brandt, Ch. Merdon, C. Manganelli, P. Farrell, Strain distribution in zincblende and wurtzite GaAs nanowires bent by a one-sided (In, Al)As shell: Consequences for torsion, chirality, and piezoelectricity, Preprint no. 3141, WIAS, Berlin, 2024, DOI 10.20347/WIAS.PREPRINT.3141 .
Abstract, PDF (31 MByte)
We present a finite-strain model that is capable of describing the large deformations in bent nanowire heterostructures. The model incorporates a nonlinear strain formulation derived from the first Piola-Kirchhoff stress tensor, coupled with an energy functional that effectively captures the lattice-mismatch-induced strain field. We use the finite element method to solve the resulting partial differential equations and extract cross- sectional maps of the full strain tensor for both zincblende and wurtzite nanowires with lattice-mismatched core and one-sided stressor shell. In either case, we show that the bending is essentially exclusively determined by $varepsilonzz$. However, the distinct difference in shear strain has important consequences with regard to both the mechanical deformation and the existence of transverse piezoelectric fields in the nanowires.
 Talks, Poster
Talks, Poster
-
A. Glitzky, Analysis of a drift-diffusion model for perovskite solar cells, 94th Annual Meeting of the International Association of Applied Mathematics and Mechanics (GAMM 2024), Session 14.07 ``Various Topics in Applied Analysis'', March 18 - 22, 2024, Otto-von-Guericke-Universität Magdeburg, March 21, 2024.
 External Preprints
External Preprints
-
B. Spetzler, D. Abdel, F. Schwierz, M. Ziegler, P. Farrell, The role of mobile point defects in two-dimensional memristive devices, Preprint no. arXiv:2304.06527, Cornell University, 2023, DOI 10.48550/arXiv.2304.06527 .
Abstract
Two-dimensional (2D) layered transition metal dichalcogenides (TMDCs) are promising memristive materials for neuromorphic computing systems as they could solve the problem of the excessively high energy consumption of conventional von Neumann computer architectures. Despite extensive experimental work, the underlying switching mechanisms are still not understood, impeding progress in material and device functionality. This study reveals the dominant role of mobile defects in the switching dynamics of 2D TMDC materials. The switching process is governed by the formation and annihilation dynamics of a local vacancy depletion zone. Moreover, minor changes in the interface potential barriers cause fundamentally different device behavior previously thought to originate from multiple mechanisms. The key mechanisms are identified with a charge transport model for electrons, holes, and ionic point defects, including image-charge-induced Schottky barrier lowering (SBL). The model is validated by comparing simulations to measurements for various 2D MoS2-based devices, strongly corroborating the relevance of vacancies in TMDC devices and offering a new perspective on the switching mechanisms. The insights gained from this study can be used to extend the functional behavior of 2D TMDC memristive devices in future neuromorphic computing applications.


