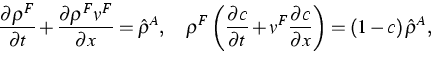
|
|
|
[Contents] | [Index] |
Cooperation with: T. Wilhelm (Universität Innsbruck, Austria)
Description: Aims and results of the project
The main aim of the project is the linear stability analysis of various flow processes coupled with the deformation of the saturated skeleton made of a poroelastic material. During the last two years three problems have been investigated:
It has already been reported that it is necessary to introduce a contribution that be nonlinear with respect to the relative velocity of components to the source of momentum in order to describe a threshold to the fluidized state of a granular material. This result has been well-confirmed by experiments performed at the University of Innsbruck (Austria).
The problem of stability of a 1-d flow through a saturated poroelastic material has been divided into two parts.
In the first part the steady state solution has been disturbed by a dynamical disturbance in the longitudinal direction. Such a flow through the immobile skeleton is described by the following field equations ([2])
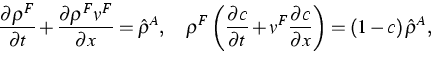
![\begin{displaymath}
\hat{\rho}^{A}:=-\rho _{ad}^{A}\frac{d\xi }{dt},\quad \frac{...
...}\left[ \frac{cp^{F}}{p_{0}}\left( 1-\xi \right) -\xi \right] ,\end{displaymath}](../2001/img422.gif)
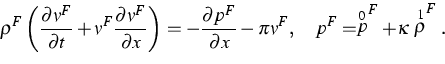 |
(1) |
The first two equations are, of course, mass balance equations for the fluid
component and for the adsorbate in the fluid. The concentration of the
adsorbate is denoted by c. ![]() is the microstructural variable called
the number of occupied sites.
is the microstructural variable called
the number of occupied sites. ![]() are constants. We consider two cases. The first one is the longitudinal
disturbance without mass exchange (i.e.
are constants. We consider two cases. The first one is the longitudinal
disturbance without mass exchange (i.e. ![]() ). In the
second one the adsorption in the nonsteady disturbance is different
from zero.
). In the
second one the adsorption in the nonsteady disturbance is different
from zero.
The base steady state flow in a pipe is described for both cases by the following relations:
The longitudinal disturbance may or may not lead to the exchange of mass between the fluid as a carrier of an adsorbate and the skeleton.
The main result of this work is that the flow (2) is stable with
respect to any such disturbance. However, the analysis leads as well to some
results on the relaxation properties of longitudinal disturbances related to
different values of permeability coefficients ![]() and
and ![]() . We
demonstrate these results in the two figures below.
. We
demonstrate these results in the two figures below.
In Fig. 2 we show similar results under the presence of mass exchange.
Qualitatively the changes in comparison to the previous case are not very
big even though there appear additional plateaus. However, quantitatively the
relaxation becomes much slower and it depends on the relaxation time of
adsorption ![]() .
.
The results for transversal disturbance of the same steady state flow are
still preliminary. We consider the 2-d flow through a channel of the width 2b. The boundary conditions in the direction of the channel are the same as
before. The boundary conditions in the transversal direction are: ![]() , where vzF is the transversal
component of the fluid velocity.
, where vzF is the transversal
component of the fluid velocity.
The flow becomes unstable in the range of high values of the bulk
permeability ![]() . The exchange of stability (
. The exchange of stability (![]() , where
, where ![]() is the complex frequency of the transversal disturbance) appears
for the values of
is the complex frequency of the transversal disturbance) appears
for the values of ![]() which grow with the growing surface permeability
which grow with the growing surface permeability ![]() . This problem shall be further investigated in the next year.
. This problem shall be further investigated in the next year.
References:
|
|
|
[Contents] | [Index] |