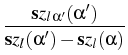[Next]:  Micro-macro transitions via modulation theory  
 [Up]:  Projects  
 [Previous]:  A study on the Becker/Döring model  
 [Contents]   [Index] 

Changes of the morphology during phase transitions

Collaborator: W. Dreyer, F. Duderstadt, B. Wagner

Cooperation with: A. Münch (Humboldt-Universität zu Berlin, Heisenberg Fellow at WIAS), W.H. Müller (Technische Universität Berlin), T. Hauck (Motorola GmbH, München)

Supported by: DFG: Priority Program ``Analysis, Modellbildung und Simulation von Mehrskalenproblemen'' (Analysis, modeling and simulation of multiscale problems)

Description:

Under this topic, we study phase transitions that generate drastic changes of the distribution of the participating phases. In particular, we were interested in unwanted coarsening phenomena and Ostwald ripening in solder materials which are used in microelectronic devices. These phenomena are triggered by surface tension and mechanical stress deviators in the bulk. Among the investigated materials were in the past the eutectic tin/lead alloy (SnPb), which is today still the most important solder material. However, due to environmental reasons, the silver/tin (AgSn) alloy will probably substitute the SnPb alloy from next year on.

Regarding the evolution of precipitates in an eutectic SnPb alloy, we have improved the boundary integral formulation of the sharp interface limit to the phase field model.

In summary, the complete boundary integral formulation for the evolution of the precipitates is:

-   $\displaystyle {\textstyle\frac{1}{2}}$$\displaystyle \Phi_{k}^{}$($\displaystyle \alpha$,$\displaystyle \tau$) + $\displaystyle {\frac{{1}}{{2\pi}}}$$\displaystyle \sum^{N}_{{l=1}}$$\displaystyle \int^{{2\pi}}_{{0}}$$\displaystyle \Phi_{l}^{}$($\displaystyle \alpha^{{\prime}}_{}$,$\displaystyle \tau$)  $\displaystyle \Im$$\displaystyle \left(\vphantom{\frac{{{\bf s} z}_{l {\alpha^{\prime}}}({\alpha^{\prime}})}{{{\bf s} z}_l({\alpha^{\prime}})-{{\bf s} z}_l({\alpha})}}\right.$$\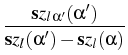displaystyle {\frac{{{{\bf s} z}_{l {\alpha^{\prime}}}({\alpha^{\prime}})}}{{{{\bf s} z}_l({\alpha^{\prime}})-{{\bf s} z}_l({\alpha})}}}$$\displaystyle \left.\vphantom{\frac{{{\bf s} z}_{l {\alpha^{\prime}}}({\alpha^{\prime}})}{{{\bf s} z}_l({\alpha^{\prime}})-{{\bf s} z}_l({\alpha})}}\right)$d$\displaystyle \alpha^{{\prime}}_{}$ + $\displaystyle {\frac{{1}}{{L_k}}}$$\displaystyle \sum^{N}_{{l=1}}$$\displaystyle \int^{{2\pi}}_{{0}}$$\displaystyle \Phi_{l}^{}$($\displaystyle \alpha^{{\prime}}_{}$,$\displaystyle \tau$)  d$\displaystyle \alpha^{{\prime}}_{}$  
+   $\displaystyle \sum^{N}_{{l=1}}$Alln($\displaystyle \bf s$zl($\displaystyle \alpha$) - Ml) = $\displaystyle \mu_{1}^{}$$\displaystyle \vert_{{\Gamma}}^{}$ , (1)
    $\displaystyle \int^{{2\pi}}_{{0}}$$\displaystyle \Phi_{k}^{}$($\displaystyle \alpha^{{\prime}}_{}$,$\displaystyle \tau$)  d$\displaystyle \alpha^{{\prime}}_{}$ = 0        k = 1,..., N - 1 , (2)
    V$\scriptstyle \bf s$$\scriptstyle \nu$k($\displaystyle \alpha$,$\displaystyle \tau$) = - $\displaystyle {\frac{{1}}{{L_k}}}$$\displaystyle \sum^{N}_{{l=1}}$$\displaystyle \int^{{2\pi}}_{{0}}$  $\displaystyle \Phi_{{l {\alpha^{\prime}}}}^{}$($\displaystyle \alpha^{{\prime}}_{}$,$\displaystyle \tau$)  $\displaystyle \Re$$\displaystyle \left(\vphantom{\frac{{{\bf s} z}_{l {\alpha}}({\alpha})}{{{\bf s} z}_l({\alpha^{\prime}})-{{\bf s} z}_l({\alpha})}}\right.$$\displaystyle {\frac{{{{\bf s} z}_{l {\alpha}}({\alpha})}}{{{{\bf s} z}_l({\alpha^{\prime}})-{{\bf s} z}_l({\alpha})}}}$$\displaystyle \left.\vphantom{\frac{{{\bf s} z}_{l {\alpha}}({\alpha})}{{{\bf s} z}_l({\alpha^{\prime}})-{{\bf s} z}_l({\alpha})}}\right)$d$\displaystyle \alpha^{{\prime}}_{}$ , (3)

together with $ \sum^{N}_{{k=1}}$  Ak = 0  . Here, Ak $ \in$ $ \mathbb {R}$, $ \Phi$ $ \in$ $ \mathbb {C}$, and the complex number Mk $ \in$ $ \Omega_{k}^{}$ being the interior of the k-th precipitate.

This set of (integral) equations provides the normal velocity of the interfaces $ \Gamma_{k}^{}$ for each of the interfaces k = 1,..., N, which is then used to evolve the interface (parametrized by $ \bf s$zk($ \alpha$)) according to

$\displaystyle {\frac{{\partial s_{k {\alpha}}}}{{\partial \tau}}}$ = V$\scriptstyle \bf t$k $\scriptstyle \alpha$ - $\displaystyle \theta_{{k {\alpha}}}^{}$V$\scriptstyle \bf s$$\scriptstyle \nu$k        and        $\displaystyle {\frac{{\partial \theta_k}}{{\partial \tau}}}$ = $\displaystyle {\frac{{V^{{{\bf s}\nu}}_{k {\alpha}}+V^{{\bf t}}_k\theta_{k {\alpha}}}}{{s_{k {\alpha}}}}}$ .     (4)

Here, $ \bf s$$ \nu_{{k}}^{{}}$ and $ \bf s$tk denote the normal and tangent vectors w.r.t. the k-th precipitate. We follow [3] for the choice of the coordinate system, where sk $\scriptstyle \alpha$ is |$ \bf s$zk|, and $ \theta_{k}^{}$ is the angle of the tangent vector at points on $ \Gamma_{k}^{}$ w.r.t. the x-axis, and Lk denotes the length of the corresponding interface.

The tangential component V$\scriptstyle \bf t$k of d$ \bf s$zk/d$ \tau$ remains arbitrary and a special choice of the parametrization for the boundaries $ \Gamma_{k}^{}$ will be used to simplify the numerical implementation. As it turns out, one can find a special choice for V$\scriptstyle \bf t$k that yields the equal arclength parametrization sk $\scriptstyle \alpha$ = Lk($ \tau$)/2$ \pi$  for all $ \alpha$, and hence the simpler ODE-PDE system

$\displaystyle {\frac{{\partial L_k}}{{\partial \tau}}}$ = - $\displaystyle \int_{{0}}^{{2\pi}}$$\displaystyle \theta_{{k {\alpha^{\prime}}}}^{}$  V$\scriptstyle \bf s$$\scriptstyle \nu$k  d$\displaystyle \alpha^{{\prime}}_{}$        and        $\displaystyle {\frac{{\partial \theta_k}}{{\partial \tau}}}$ = $\displaystyle {\frac{{2\pi}}{{L_k}}}$$\displaystyle \left(\vphantom{V^{{{\bf s}\nu}}_{k {\alpha}}+\theta_{k {\alpha}}\;V^{{\bf t}}_k }\right.$V$\scriptstyle \bf s$$\scriptstyle \nu$k $\scriptstyle \alpha$ + $\displaystyle \theta_{{k {\alpha}}}^{}$  V$\scriptstyle \bf t$k$\displaystyle \left.\vphantom{V^{{{\bf s}\nu}}_{k {\alpha}}+\theta_{k {\alpha}}\;V^{{\bf t}}_k }\right)$  .     (5)

It turns out that there are three derivatives in the evolution equation for $ \theta_{{k {\alpha}}}^{}$. Such high derivatives will lead to numerical stiffness (the stability constraint, e.g.,   $ \bigtriangleup$   t < O$ \left(\vphantom{({\hspace{0em}\bigtriangleup\hspace{-0.1em}} x)^3}\right.$(   $ \bigtriangleup$   x)3$ \left.\vphantom{({\hspace{0em}\bigtriangleup\hspace{-0.1em}} x)^3}\right)$, leads to prohibitive time stepping). However, the advantage of the above equal arclength formulation with a special choice of V$\scriptstyle \bf t$k is that the evolution equation for each $ \theta_{k}^{}$ can be written in Fourier space as

- $\displaystyle \left(\vphantom{\frac{2\pi}{L}}\right.$$\displaystyle {\frac{{2\pi}}{{L}}}$$\displaystyle \left.\vphantom{\frac{2\pi}{L}}\right)^{3}_{}$|j$\displaystyle \vert^{3}_{}$  $\displaystyle \theta$ + $\displaystyle \hat{N}$($\displaystyle \alpha$,$\displaystyle \tau$), (6)
where the first term contains the high derivative and hence is the stiffest, so we treat it implicitly. In this form it is linear and diagonal in Fourier space and hence one only has to solve a diagonal system. It can be shown that the nonlinear expression $ \hat{N}$ can be treated explicitly. We use a pseudospectral method (using FFT) in space, and leap-frog for the explicit and Crank-Nicholson for the implicit time integration. The integration of the L$\scriptstyle \tau$ ODE is done with an Adams-Bashforth integrator. Equations (1), (2), (3), and $ \sum^{N}_{{k=1}}$  Ak = 0 yield $ \Psi$ and Ak. They represent a linear system which is solved iteratively using GMRES.

Some first studies have appeared in [2] with just two precipitates. We also used this method to simulate the coarsening behavior of larger sets of precipitates and are presently deriving the sharp-interface model including mechanical effects for implementation into our code.

The change of the material and type of applications enforces several changes to the current model, which was originally designed to study the binary tin/lead alloy with two coexisting disordered phases.

For example, if eutectic SnPb is brought in contact with a copper plate, there results the ordered phase Cu5Sn7. An ordered phase is also present in AgSn. In both cases, the concentration variable of a binary disordered mixture is not sufficient anymore to indicate which phase is present at a local space point.

The Grinfeld instability whereupon a plane interface might become unstable if mechanical stress deviators are involved, becomes increasingly more important. We have started a study on this subject with two first objectives: (i) Establishment of a rational procedure to derive the sharp-interface equations that describe the Grinfeld instability; (ii) Examination of the influence of boundary conditions on the onset of the instability.

References:

  1. W. DREYER, A. MÜNCH, B. WAGNER, On the Grinfeld instability, to appear as WIAS Preprint.

  2. W. DREYER, B. WAGNER, Sharp-interface model for eutectic alloys. Part I: Concentration dependent surface tension, WIAS Preprint no. 885, 2003 , to appear in: Interfaces Free Bound.

  3. H.J. HOU, J.S. LOWENGRUB, M.J. SHELLEY, Removing the stiffness from interfacial flows with surface tension, J. Comput. Phys., 114 (1994), pp. 312-338.


 [Next]:  Micro-macro transitions via modulation theory  
 [Up]:  Projects  
 [Previous]:  A study on the Becker/Döring model  
 [Contents]   [Index] 

LaTeX typesetting by H. Pletat
2005-07-29