A study on the Becker/Döring model

[Next]:

Changes of the morphology during phase

[Up]:

Projects

[Previous]:

Numerical analysis of surface waves on

[Contents]

[Index]

A study on the Becker/Döring model

Collaborator: W. Dreyer, F. Duderstadt

Cooperation with: B. Niethammer (Humboldt-Universität zu Berlin), M. Jurisch, S. Eichler (Freiberger Compound Materials GmbH (FCM)), P. Rudolph, F. Kießling (Institut für Kristallzüchtung, Berlin), K. Haack (GTT-Technologies, Herzogenrath)

Description:

The Becker/Döring (BD) model describes processes where a droplet with $\alpha$ atoms may grow by incorporation of a single atom from the surroundings and shrink by emitting a single atom into the surroundings. Other processes, like the appearance of a droplet with $\alpha$ + $\beta$ atoms by the reaction of a droplet with $\alpha$ > 1 atoms with another droplet with $\beta$ > 1 atoms, are not considered within the BD model.

The transition rates of the two processes give the number of reactions per second, and they are denoted by $\Gamma_{{\alpha}}^{{E}}$ and $\Gamma_{{\alpha}% }^{{C}}$ . Their derivation for the case of precipitation of liquid droplets in GaAs is one of the objectives of this study.

We consider a distribution of droplets with $\alpha$ $\in$ {1, 2,..., $\nu$ } atoms and we introduce a set of functions Z(t, $\alpha$ ) $\geq$ 0, which give at any time t $\geq$ 0 the number of droplets with $\alpha$ atoms. The number of single atoms is included here and it is given by Z(t, 1). The choice of the largest considered droplet with $\nu$ atoms is a subtle problem, which is not discussed here.

The evolution of Z(t, $\alpha$ ) is determined by a system of ordinary differential equations that we call nowadays the BD system. For a thermodynamic system with a fixed number of atoms, it reads

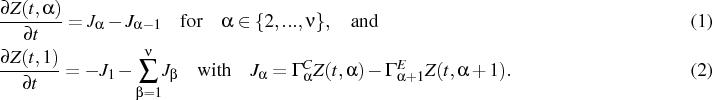
$\begin{align} & \left. \frac{\partial Z(t,\alpha)}{\partial t}=J_{\alpha}-J_{\al... ...ha}^{C}Z(t,\alpha )-\Gamma_{\alpha+1}^{E}Z(t,\alpha+1).\right. \quad \end{align}$
Equilibrium is established for J = 0, and a thermodynamic treatment provides the distribution of droplets in equilibrium by minimizing the available free energy of the system

$\displaystyle \mathfrak{A}=kT{\displaystyle\sum\limits_{\beta=1}^{\nu}} Z(t,\be... ...beta)\quad\text{and}\quad q_{\alpha}=\exp(-\frac{\mathcal{A}_{\alpha}% }{kT}).$

(3)

Here, N_D denotes the total number of droplets and $\mathcal {A}$ is the available free energy of the system containing a single droplet with $\alpha$ atoms.

One of the important results which were obtained during the period of this report regards the observation that the transition rates $\Gamma_{{\alpha}% }^{{E}}$ and $\Gamma_{{\alpha}}^{{C}}$ are not independent from each other because we have proved the

Theorem: A sufficient condition that the BD system implies the existence of a Lyapunov function that can be identified with $\mathfrak{A}$ reading

$\displaystyle {\frac{{\Gamma_{\alpha+1}^{E}}}{{\Gamma_{\alpha}^{C}}}}$ = $\displaystyle {\frac{{N_{D}}}{{Z(t,1)}% }}$ $\displaystyle {\frac{{q_{\alpha}}}{{q_{\alpha+1}}}}$ .

(4)

This condition is violated in all studies of the BD system that can be found in the literature.

References:

W. DREYER, F. DUDERSTADT, On the Becker/Döring theory of nucleation of liquid droplets in solids, WIAS Preprint no. 997, 2004 .
, On the modeling of semi-insulating GaAs including surface tension and bulk stresses, WIAS Preprint no. 995, 2004 .
W. DREYER, F. DUDERSTADT, S. QAMAR, Diffusion in the vicinity of an evolving spherical arsenic droplet, WIAS Preprint no. 996, 2004 .